Basic Electrical Engineering Questions and Answers
Q1.
Find the value of the currents I1 and I2 flowing clockwise in the first and second mesh respectively.
a) 0.96A, 1.73A
b) 0.96A, -1.73A
c) -0.96A, 1.73A
d) -0.96A, -1.73A
Ans: b
The two mesh equations are:
5I1-3I2=10
-3I1+7I2=-15
Solving the equations simultaneously,
we get I1=0.96A and I2=-1.73A.
2.
Find the value of V if the current in the 3 ohm resistor=0.
a) 3.5V
b) 6.5V
c) 7.5V
d) 8.5V
Ans: c
Taking the mesh currents in the three meshes as I1, I2 and I3, the mesh equations are:
3I1+0I2+0V=5
-2I1-4I2+0V=0
0I1+9I2+V=0
Solving these equations simultaneously and taking the value of I2=0, we get V=7.5V.
3.
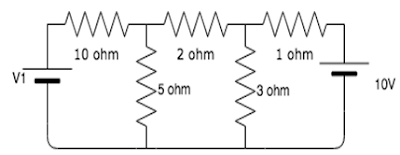
Find the value of V1 if the current through the 1 ohm resistor=0A.
a) 83.33V
b) 78.89V
c) 87.87V
d) 33.33V
Ans: a
Taking I1, I2 and I3 as the currents in the three meshes and taking I3=0 since it is the current across the 1 ohm resistor, the three mesh equations are:
15I1-5I2=V1
-5I1+10I2=0
3I2=10
Solving these equations simultaneously we get
V1= 83.33V.
4.
Calculate the mesh currents I1 and I2 flowing in the first and second meshes respectively.
a) 1.75A, 1.25A
b) 0.5A, 2.5A
c) 2.3A, 0.3A
d) 3.2A, 6.5A
Ans: a
In this circuit, we have a super mesh present.
Let I1 and I2 be the currents in loops in clockwise direction. The two mesh equations are:
I2-I1=3
-5I1-3I2=5
Solving these equations simultaneously, we get I1 = -1.75A and I2 = 1.25A.
Since no specific direction given so currents in loop 1 and loop 2 are 1.75A and 1.25A respectively.
5.
I1 is the current flowing in the first mesh. I2 is the current flowing in the second mesh and I3 is the current flowing in the top mesh. If all three currents are flowing in the clockwise direction, find the value of I1, I2 and I3.
a) 7.67A, 10.67A, 2A
b) 10.67A, 7.67A, 2A
c) 7.67A, 8.67A, 2A
d) 3.67A, 6.67A, 2A
Ans: a
The two meshes which contain the 3A current is a super mesh. The three mesh equations therefore are:
I3=2A
I2-I1=3
-2I1-I2=-26
Solving these equations simultaneously we get:
I1=7.67A, I2=10.67A and I3=2A.
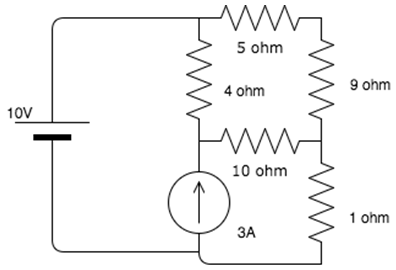
6.
Calculate the mesh currents.
a) 7A, 6A, 6.22A
b) 2A, 1A, 0.57A
c) 3A, 4A, 5.88A
d) 6A, 7A, 8.99A
Ans: b
The two meshes which contain the 3A source, act as a supper mesh. The mesh equations are:
I1-I3=-3
4I1-14I2+11I3=10
4I1-28I2+10I3=0
Solving these equations simultaneously, we get the three currents as
I1=-1A, I2=0.57A, I3=2A
So currents are 2A, 1A, 0.57A.
7.
Find the value of the node voltage V.
a) -60V
b) 60V
c) 40V
d) -40V
Ans: a
The node equation is:
-2+8+V/10=0 => 6 + v/10 = 0 => v = -10*6 = -60V
Solving this equation, we get V = -60V.
8.
Calculate the node voltages V1 and V2.
a) 12V, 13V
b) 26.67V, 11.33V
c) 11.33V, 26.67V
d) 13V, 12V
Ans: c
The nodal equations are:
2V1-V2=-4
-4V1+5V2=88
Solving these equations simultaneously,
we get V1=11.33V and V2=26.67V.
9.
Find the node voltage V.
a) 1V
b) 2V
c) 3V
d) 4V
Ans: d
The nodal equation is:
(V-10)/2+(V-7)/3+V/1=0
Solving for V, we get V=4V.
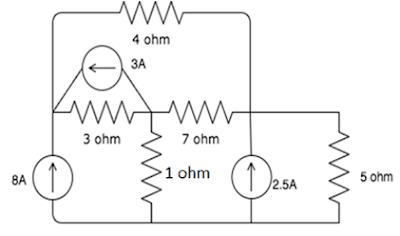
10.
Calculate the node voltages.
a) 30.77V, 7.52V, 18.82V
b) 32.34V, 7.87V, 8.78V
c) 34.34V, 8.99V, 8.67V
d) 45.44V, 6.67V, 7.77V
Ans: a
The nodal equations, considering V1, V2 and V3 as the first, second and third node respectively, are:
-8+(V1-V2)/3-3+(V1-V3)/4=0
3+V2+(V2-V3)/7+(v2-V1)/3=0
-2.5+(V3-V2)/7+(V3-V1)/4+V3/5=0
Solving the equations simultaneously,
we get V1=30.77V, V2=7.52V and V3=18.82V.













0 Comments